Disorder, Chaos, and Existence

Chaos and disorder are frequently associated with political anarchy, but this chapter is only about what order means and the confused idea that disorder means chaos or destruction and the interesting mathematical concept of chaos. Interestingly enough, there is a relationship between chaos, in the mathematical sense and the “chaos” that, supposedly, anarchy would lead to. Since that would be a digression from the subject at hand, political chaos will be fully explicated in the, “Social Chaos,” chapter.

This chapter is partly about that branch of mathematics called chaos, including fractals and strange attractors, it is not a technical discussion of that subject, but a way of introducing some related philosophical ideas having to do with disorder, existence, and the limits of mathematics.

There is some math, and there are some technical terms, but for the most part, this is not a very technical discussion.

What is Order?

What exactly is chaos? The common view, I think, is that chaos is extreme disorder, or even complete disorder. Without understand what order is, however, this description is not very helpful.

Ironically, one of the most confusing of concepts is the concept of order. The word itself has more than one meaning or connotation, and those meanings are often confused:

1.There is numerical order meaning being lined up according to a priority or hierarchy.

1. There is functional order meaning a purposeful organization or arrangement.

2. There is natural order meaning the entities and events of reality determined by the nature of material existence, including all the principles of the sciences and the nature of life, consciousness, and mind.

3. There is uniform order meaning without difference or variation.

4. There is entropic order as used in information theory and the thermodynamics.

_Numerical order_—is any order that can be identified by the ordinal number, first, second, third, fourth, etc. Numerical order is an epistemological method of identifying relationships in space or time, and by analogy relationships between values and concepts. …. first tree, second tree… first lesson of the day, second lesson of the day.. first prize, second prize, grammatical order, taxonomy order, etc……..

_Functional order_—is any order that is based on an intended purpose of a method, process, or design and may include numerical orders (a recipe for example). When any process fails to fulfill its intended purpose is a disorder. When natural events, determined by physical laws, are described as order, it is not functional order–there is no intended purpose or objective. If the event were totally different there would be no disorder.

_Natural order_—is the order of the natural world, determined by the nature of material existence. It is the order determined by the basic principles of ontology and necessity of difference. Natural order is often mistaken for functional order, but natural order has no intrinsic objective or purpose.

_Uniform order_—is the converse of natural order. Natural order requires difference which makes existents possible. Uniformity is a lack of difference and essentially a anti-existent principle. _Entropic order_—is the oder of distribution of either usable energy (in thermodynamics) or data integrity (in information theory). Like uniform order it is the converse of other meanings of order. More entropy means less difference in energy or data integrity, less entropy means more difference in energy and data integrity.

Existence Requires Disorder

In the Basic Principles of Ontology the second corollary of existence is, “The Necessity of Difference—Anything that exists must be different in some way from everything else that exists. No two things can be identical in every way.” The difference must be a difference in qualities or attributes; no two existents can have exactly the same qualities.

That necessity of difference relates to chaos and existence. This could be explained by reference to thermodynamics and entropy, but this is meant to be an untechnical discussion, so I will use an illustration to explain why disorder is necessary to existence.

It is order in the “uniformity,” or “regularity,” this disorder pertains to. If we consider a hypothetical universe that is totally uniform, whatever aspect we consider, entities, attributes, or existents, total uniformity would mean no difference, everything would have be identical, have the same attributes, and behave in the same way. I use the following illustration to demonstrate that nothing could exist in such a hypothetical universe.

A Homogeneous Universe

Here is a universe which is totally uniform with no variations or disorderliness whatsoever. Whatever it consists of, it is the same everywhere (though there could be no “where” in such a universe) and all the time (though there could be no time in such a universe). Such a universe could not exist, because nothing could exist in it. The ontological reason such a universe cannot exist is that there are no differentiating attributes, nothing that can differentiate one existent from another.

………………………  
………………………  
………………………  
………………………

Even if totally different attributes are imagined for such a universe, if it is truly uniform, still nothing can exist. Ontologically, for the same reason, there are no differentiating attributes or qualities, because everything has the same attributes (which “everything” is of course “nothing.”)

###########################  
###########################  
###########################  
###########################

Even if there were differentiating attributes, if existence were distributed uniformly, there could be no existents.

#.#.#.#.#.#.#.#.#.#.#.#.#.#  
#.#.#.#.#.#.#.#.#.#.#.#.#.#  
#.#.#.#.#.#.#.#.#.#.#.#.#.#  
#.#.#.#.#.#.#.#.#.#.#.#.#.#

An uneven distribution makes difference possible. With just two attributes, not uniformly distributed, there can be at least two things, an “###…” thing and “……” thing.

###########################  
###########################  
………………………  
………………………

A universe with only two things in it is not much of a universe (an impossible one actually). A real universe must have many different kinds of differences, enough for every different existent there is. This universe for example has many different existents, #, ##, ###, ####, etc.

.#…..#..####..####..#….  
.#..#…..#…..#…..#..##  
#…###…#…..#..#…..#  
. ….####..#..#…..####…

Just by looking at that universe in a different way, it can be seen it even has “life” in it. (The two illustrations above and below have exactly the same number and disorder of #s in them.)

.#…..#..####..####.  
.#…..#..#…..#….  
.#…..#..###…###..  
.#…..#..#…..#….  
.####..#..#…..####….

The entire point of this illustration is to emphasize the necessity of disorder, in the sense of non-uniformity and irregularity, to existence. The last “life” illustration illustrated two things I cannot elaborate on here. The first is that order is logically first, organization, logically second. Only organization that does not reduce disorder, that is, organization that increases difference is “creative.” Organization the reduces disorder, that is, that decreases difference, is destructive.

The second is that some organization only reveals relationships that otherwise are not apparent or recognizable. Conceptualization is one example of such organization. The most common one, is counting.

The Universe is Not Orderly

The universe is not orderly, it is chaotic, and if it were orderly it could not exist. It is difference and change that make everything possible, and both are disorderly.

The disorderliness of the universe is neither random or haphazard. It is a determined disorder. If it were not for this disorder, the so called heat death of the universe would be assured. In fact, that heat death is probably not possible because most of the behavior of the universe is not, in the normal sense, geometric or linear. While heat does not violate the first law of thermodynamics, entities and substances are not restricted by that law, and since it is entities in which heat is distributed, (the existence of infrared, for example, is only known if some body is heated by it).

In a sense, Maxwell’s demon exists, and its name is Fractal Chaos. Nothing physical can violate the second law of thermodynamics, and if the universe were orderly and uniform it’s heat death would be asured—it is no orderly and uniform, however, and has a tendency to “clump up” and distribute itself in unpredictable ways. While heat is distributed evenly, the matter in which it is manifest is not, which assures, on a cosmic scale, heat itself can never be uniformly distributed.

Determined But Not Predictable

There is a branch of mathematics called “chaos” or “fractals” or “Lorenz attractors.” Most events have some elements of behavior that are described by that kind of mathematics. While most people have heard of the esoteric “Julia,” “Mandelbrot,” and possibly the, “Lyapunov,” sets, and my have seen images produced to represent them, they have no idea what they are.

Most of the explanations of fractals and chaos are in mathematical terms, and those without a mathematical background find those explanations next to useless. In fact, they are not terribly difficult to understand and the problem is, mathematicians are not very good at explaining.

The mathematics of chaos is actually an approximation of the natural phenomena they reflect. In reality, the chaotic behavior has the same kind of determined but unpredictable nature as fractals, but fractal math is discrete and natural chaos is analog. Some early experiments with Lorenz attractors, used analog computers for modeling things like the weather and fluid dynamics. Today, digital computers approximate the analog functions by means of equations with discrete outputs.

What Is a Fractal Equation

If you’ve ever read anything about “fractal mathematics,” it usually begins talking about “functions”and “functions of functions” and is filled with symbols like, f(f(f(x))). Unless you are at least somewhat familiar with the concepts of the Calculus, you are lost already.

In fact, the concepts of fractals are much simpler than such writers imply. They are, however, mathematical in nature, and if such concepts as “squares” (a number multiplied by itself) and “equations” (such as a + b = c) are vague, the mathematical nature of fractals still might evade you. I will, however, provide a non-mathematical explanation following the mathematical one.

Fractal Math

Fractals are called “iterative,” which only means, a particular equation is repeated over and over. In fractal math, the “output” of each iteration of an equation, is used as one of the input variables of the equation for the next iteration.

For example, _x_2 + 𝑐 = x`, (the basic equation for both Mandelbrot and Julia sets, if you are familiar with them) simply means x “squared” (or x times x), plus 𝑐 equals x`. The value of 𝑐, called a variable, is “fixed”(if a Julia set) but varies (if a Mandelbrot set). The right side of the equation (x’) is the value of x to be used in the next iteration.

This is how it works. Assume the beginning value of x is 0, and the value of 𝑐 is 3. If we plug those values into our equation we have 02 + 3 = x’ the result is 3. (02 + 3 = 3). The next iteration uses the result x’ (3) as the input value of “x” in the next iteration. Therefore, the next iteration is 32 + 3 = x’, and the result is 12 (32 + 3 = 12). Since 12 is the new value of x for the next iteration, the equation will be 122 + 3, or 147. The next iteration will be 1472 +3, ….well you can see this thing just keeps getting bigger and bigger. Two aspects of fractals are already apparent, however, no number ever repeats, and the results cannot be predicted, except in this case it can be predicted the numbers keep getting bigger.

A better example is (x-𝑐)/x = x‘ [x\=1, 𝑐\=3, x` is the value of x for the next iteration]

The first iteration is therefore: (1-3)/1 = x’, or -²⁄₁= -2. The series begins as follows:

-²⁄₁ = -2
-5/-2 = 2.5
-.⁵⁄₂.5 = -.2
-3.2/-.2 = 16
¹³⁄₁₆ = .8125
-2.1875/.8125 = -2.692307
-.³⁰⁷⁶⁹²⁄₂.692307 = -.114285
-3.114285/-.114285 = .355918
3.355918/.355918 = 9.428899
6.⁴²⁸⁸⁹⁹⁄₉.428899 = .681829
-2.318170/.681829 = -3.399928

The output of all equations produces a series of values: -2, 2.5, -.2, 16, .8125, -2.692307, -.114285, .355918, 9.428899, .681829, -3.399928, …. (All values have been truncated to 6 decimal places.) The series is indefinite and never repeats. Notice that the change in signs seems to be random. It is not random, because it is determined by the equation and input values, but cannot be predicted. In fact, no part of the series can be predicted.

Given the same starting values for 𝑐 and x, however, the identical series is always produced. In fact, if any value of x at any point in the series is used as the starting value, the series will continue from that point just as if the whole series were produced.

It is impossible to determine the starting values or equation from any portion of a fractal series, and even if the equation is known, the starting values cannot be determined.

Most importantly, the values seem to be random. A series like the one above has no obvious or apparent pattern. Nevertheless, it is determined, and if plotted by a computer program designed to make a fractal series, “visible,” like the images illustrating this article, it is obvious there is a pattern with the parts that seem to repeat themselves, but in fact, no two parts are ever identical.

The following link is to a resource for anyone who would like to learn more about the history, the mathematics, and the application of chaos and fractals: Mathematics and the “Fractional”Dimension

I Did Not Forget

I promised to provide a non-technical or non-mathematical description of chaos. There are actually a number of such descriptions, but most do not give a good sense of the significance of chaos. There are many aspects of the real world that are examples of chaos. The human heart beat, for example, is never absolutely even, because the electronic nature of the heart behaves, apparently, like a Lorenz attractor. It is a feedback mechanism (like taking the output of one equation and using it as the input of the next). In fact, if the heartbeat were perfectly symmetrical, it would race uncontrollably, a condition which does happen called fibrillation.

Snowflakes are examples of fractals. Each is completely different, because the physics that forms them, though identical, begins with a different value for each snowflake (because the particles of dust all snowflakes form on are slightly different).

Ferns are another an example. While ferns all look very similar, they are never identical. Broccoli exhibits the same fractal characteristics. There are, in fact, chaotic characteristics in all life. The venous and arterial systems in a human kidney, flowers, and trees are all examples, and DNA clusters form shapes that resemble Julia sets. Non-living examples include clouds, frost and ice formations, lightning, and galaxies.

Perhaps the most interesting example of chaos examples is the weather. It was while studying weather that the famous Lorenz attractor was discovered. Edward Lorenz, an MIT meteorologist was attempting to create a program that all meteorologists dream of. It was believed if one could map all the meteorological states of the world, one cold predict all the weather, indefinitely. What they discovered was, because weather “feeds itself” it behaves chaotically, and therefore was unpredictable, which came as no surprise to anyone except meteorologists

These examples demonstrate that chaos is part of almost every aspect of physical existence and its behavior but do not explain how it works. For that an illustration is needed.

A Chaotic Water Wheel

Edward Lorenz designed this simple mechanism to demonstrate chaotic behavior non-mathematically.

The device is a water wheel with buckets mounted on swivels. There is a small hole at the bottom of each bucket. Some friction is intentionally introduced to prevent the wheel from being completely free-wheeling.

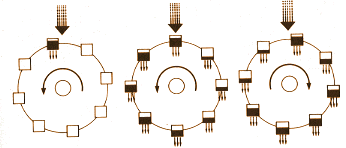
The water is poured into the buckets from a spout at the top. If the water is poured slowly, it will trickle out of the hole in the bottom and not enough force is generated to overcome the friction and the wheel remains stationary. If the flow of water is increased, the wheel will begin to rotate. As it accelerates, each bucket receives less water until eventually the first buckets filled are heavier than the last buckets filled. When the heavier buckets begin to ascend on the opposite side of the wheel, they eventually outweigh the buckets on the other side, and the wheel changes direction.

The process begins in the opposite direction, but now there are partially filled (and continuously emptying) buckets ascending on the opposite side to those being filled and descending. The next reversal can therefore take much longer to occur (if the buckets on the other side slow the wheel enough for descending buckets to fill more) or very quickly, (if the buckets on the other side do not slow the wheel, and the descending buckets fill less).

The speed of the wheel at any time, it’s direction, and when reversals will occur cannot be predicted. The behavior is totally chaotic. It is truly chaos in the sense that the only input is constant (the flow of water) and the behavior to that input is determined by the mechanisms own behavior, because it is its own rate of rotation and reversals that determine how much each bucket will be filled at any time.

See it in action here.

Limits of Mathematics

There is in many people, maybe even most, an overwhelming desire for some one single ultimate answer or explanation for everything. That desire is born of an irrational fear of the unknown that I will address in another place, and it manifests itself in various ways. For some, the ultimate answer for everything is God, for others it is the illusive Grand Unified Theory (GUT) of physics. All such ultimate answers, however, are really a kind of mysticism, and those who accept some supposed ultimate answer, whether God, or GUT, or something else, embrace it with religious fervor.

Perhaps the most fervent of “true believers,” are those who embrace what I call the Pythagorean superstition. It is the belief that numbers or mathematics is the ultimate answer to everything, or the only true means to it.

Pythagoras said, “all things are numbers.” Modern Pythagoreans do not say all things are numbers, but do believe everything can ultimately be understood in terms of numbers or explained by mathematics. When the ancient pythagoreans discovered incommensurables, some of them committed suicide, because that discover showed that all they believed, the very basis of meaning in their lives, was wrong. I hope the modern pythagoreans will not react to what I have to say with similar despair.

Two Failures of Pythagoreanism

Chaos theory has already demonstrated reality is full of chaotic behavior that mathematics is incapable of fully describing. It can describe the general nature of such behavior, but no specific chaotic event or action can be described by any specific mathematical equation or value. This is actually the second failure of Pythagoreanism. The first was that discovered by Pythagoras, himself. The disillusionment of the ancient Pythagoreans followed directly from Pythagoras’ greatest discovery that, where 𝑎 and 𝑏 are the legs (sides next to the right angle) of a right triangle, and 𝑐 its hypotenuse, (side opposite the right angle), 𝑎² + 𝑏² = 𝑐². This led immediately to the discovery that in an isosceles right triangle, where 𝑎 = 𝑏, there is no unit of measure that can measure both a leg of an isosceles right triangle and the hypotenuse.

Today many such irrational relationships are known, and very close approximations are used in calculations where such relationship need to be measured, such a pi. It is difficult not to have the impression that irrationals, like pi, actually do have a value if one could just carry it out far enough. The ancient way of describing these irrational relationships is much clearer.

Suppose the sides of an isosceles right triangle are one inch long. Let the length of the hypotenuse be represented by 𝑚/𝑛. Since 𝑎² + 𝑏² = 𝑐², substituting 1 for both 𝑎 and 𝑏, and 𝑚/𝑛 for 𝑐, yields 𝑚²/𝑛² = 2. Divide out any common factor in 𝑚/𝑛, now either 𝑚 or 𝑛 must be odd (because if both are even there is still the common factor 2).

Multiply both sides of the equation, 𝑚²/𝑛² = 2, by 𝑛² to get 𝑚² = 2𝑛². Therefore, 𝑚² is even; therefore 𝑚 is even. Suppose 𝑚 = 2𝑝 (if 𝑚 is even it must be 2 times something). Substituting 2𝑝 for 𝑚 in the equation, 𝑚² = 2𝑛², yields 4𝑝² = 2𝑛². Dividing both sides by 2 yields 𝑛² = 2𝑝², therefore 𝑛 is even.

If there were a unit of measure that could measure both a leg and hypotenuse of an isosceles right triangle, the length of the hypotenuse could be represented as 𝑚/𝑛 units, and either 𝑚 or 𝑛 would have to be odd. Since both 𝑚 and 𝑛 can be demonstrated logically (or mathematically) to be even, there can be no unit of measure that can measure both a leg and hypotenuse of an isosceles right triangle.

This discovery was enough to demonstrate to the ancient pythagoreans that not only is, “all things are numbers,” not true, all things cannot even be described by numbers. It is even worse than that, however, for the modern Pythagoreans.

Mathematically Unknowable

Modern day Pythagoreans, who are almost always physicalists, believe that everything can ultimately be explained and known in terms of mathematics. The ancient Pythagoreans discovered that this is a great mistake, a discovery modern day Pythagoreans have yet to make. Chaos theory, for those who understand it, is another piece of evidence that many things in this world cannot be known by means of mathematics—oddly, it is mathematics itself that demonstrates it just as it was mathematics that demonstrated it for the ancient Pythagoreans.

Chaos and incommensurables are only two difficulties that most modern-day pythagoreans hope can be overcome. But even if they could, Pythagoreanism would still be wrong. With a single exception, nothing measurable can ever be exactly known by means of mathematics.

The single exception is entities that can be counted. If there are sheep in a pen, the exact number of sheep can be determined exactly by counting them. It is even possible to determined the exact number of hairs on a sheep by counting them. What cannot be determined exactly, ever, is exactly how much a sheep weighs, for example, because weight requires measurement, and no measurement can ever be perfectly exact.

It might be supposed this is only because of the limitations of precision in our measuring devices. In part, this is true, but even if there were no limit to how precise we could make our instruments, we still could never make any measurement with absolute exactness. The reason is the nature of mathematics itself, the nature of measurement, the nature of reality, and the nature of concepts.

Mathematics is a method. Numbers are the invention of men, and they were invented to make it possible to count. All of mathematics is an extension of that basic method of determining the number of things by counting. Addition and subtraction are just “counting” and “counting backwards” shorcuts. Multiplication and division are addition and subtraction shortcuts. Fractions and decimals are division shortcuts and methods of notation.

Measurement is applying the method of mathematics to determining the relationship between similar attributes in entities, such as length. The observation that some things have the common attribute length but that most do not have the same length requires a method to determining the differences in various lengths. It was discovered the method of counting could be used to make that determination. By using something with some length (unit of measure), and laying it on a thing whose length was to be determined, the number of times that unit of measure could be laid, starting at the place it last ended each time, till it reached the end, would indicate the length as so many “units of measure.”

Units of measure, however, do not exist in nature. They are the invention of men, chosen for what seems practical and useful to whatever is being measured. But every unit of measure is arbitrary, and could be anything. When “counting”units of measure, there are no actual metaphysical things being counted, just some arbitrarily chosen “length” or “weight” or “time,” which must be something with length as an attribute, or “weight” as an attribute, or, “motion” as an attribute.

Units of measure are discrete, they are concepts and all concepts are discrete. But concepts have no physical existence, only mental or conceptual existence. There are no metaphysical inches, pounds, or minutes, there is only length, weight, and time, and they are all analog.

For any discrete unit of length conceived, there may be existents it can exactly measure, but there are, potentially, an infinite number of existents it cannot measure exactly. This is true of all units of measure. To suppose that everything can ultimately be known in terms of mathematics forgets that mathematics is only a method, a method for dealing with measurable attributes of existents and their relationships and is always inexact.

This in no way repudiates the value and importance of mathematics. All that men have achieved and accomplished in the fields of science and technology rests heavily on mathematics and without the knowledge of that method, all the benefits that contribute to the quality of life enjoyed by modern man in western civilization would not be possible. It is when mathematics, or any other single branch of knowledge, is raised to the level of mystic insight that will provide the answers and explanation for all things that it becomes a superstition.

—(06/07/05)